Humidity, Temperature and Condensation Control
One consequence of thermal bridging is that some surfaces can get cold enough to allow condensation of water vapour from the indoor air. The collected moisture can corrode steel, rot wood and allow mould growth. Condensation control is an important factor in the success and durability of buildings.
The term "humidity" refers to water vapour in the air. The amount of water vapour that air can hold depends on its temperature. Relative Humidity (RH) defines the ratio of actual vapour in the air to the maximum amount the air could hold at that
temperature.
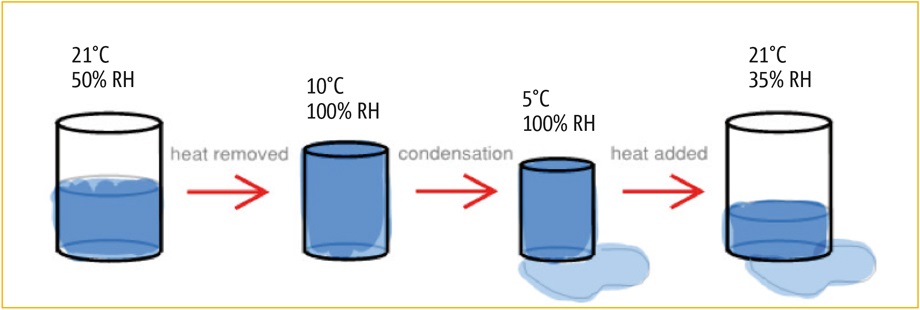
Figure 30 is an illustration explaining some of the interactions between air, vapour, temperature and liquid water. It is based on the idea that air is like a container that changes size with temperature, representing air's ability to hold moisture. If you start with air at 21°C and 50% RH, and allow the air to cool so our imaginary container shrinks, the relative humidity will increase even though there is no water added or taken away. At some point, which happens to be about 10°C, the container is full to the top. This is the point where the air is saturated and is at 100% RH. This is also called the dew point temperature.
If further cooling occurs, the container shrinks and some water spills over the top. This is the same as moisture condensing from the air as a liquid. The air is still at 100% RH but a calculable volume of liquid water has been released.
If the air is now warmed (without adding back the condensed moisture) the RH would go down, and when it reaches the same starting temperature, the air would be of a lower RH than at the beginning of the exercise.
All these relationships can be calculated using psychrometric charts and tables, but the important thing is to understand the relationships between cold surfaces, humidity and condensation:
- Air that has water vapour in it has a Dew Point Temperature: the temperature where the air is saturated.
- Condensation will form on a surface that is below the Dew Point Temperature of the air to which it is exposed.
- The more vapour in the indoor air, the higher its RH and dew point temperature. Therefore, the less cold a surface has to be to start condensing, and the more condensation forms on a surface of a given temperature below the dew point.
- The relative humidity of the air layer in contact with a cold surface will be at a higher value than the warmer air away from the surface.
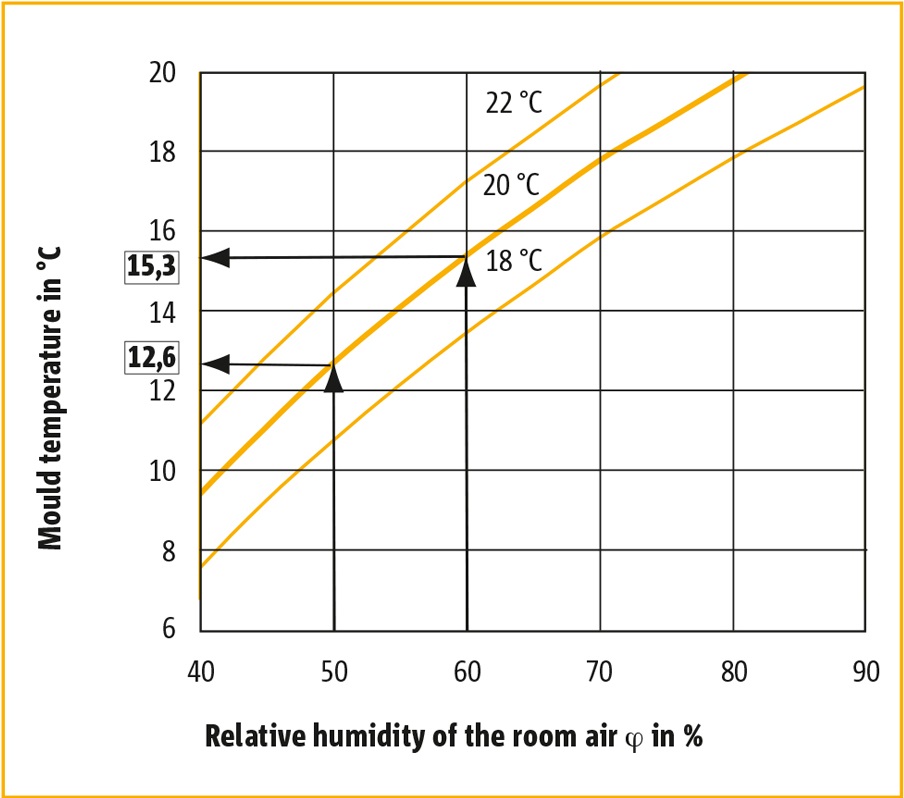
A major concern with condensation or high local RH caused by cold surfaces is that it can lead to mould growth. Because mould spores and suitable nutrients (cellulose) are virtually always present in dust, all that is required to cause mould growth in a building is a sufficient amount of moisture in the microclimate in which the spores exist. Liquid water as formed by condensation is a particular concern, but some mould species can grow in high humidity conditions without actual liquid water. In Europe the concept of "Mould Temperature" is used which is similar to the Dew Point Temperature, but defines the temperature where air is at 75% RH. Figure 31 and 32 compare the Dew Point Temperature and Mould Temperature for a range of indoor conditions.
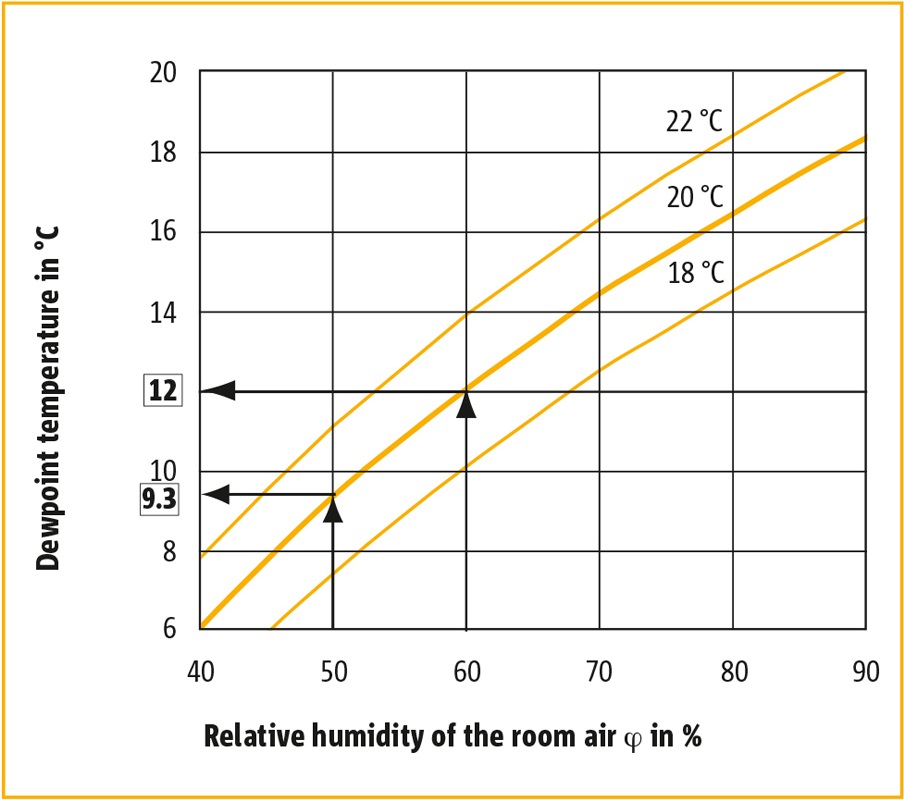
The dew point temperature is dependent upon the inside air temperature and humidity (see Figure 18). The higher the relative inside air humidity and temperature, the higher the dew point temperature becomes, meaning the sooner condensation begins to form on cold surfaces.
The indoor air climate is typically an average of approx. 20 °C and 50% relative humidity. This puts the dew point temperature at 9.3 °C. Rooms frequently exposed to moisture, such as bathrooms, can easily reach higher humidity levels of 60% or more. This also raises the dew point temperature and increases the risk of condensation forming. At a humidity level of 60% in a room, the dew point temperature is already at 12.0 °C.
Mould growth on building component surfaces can occur with moisture levels as low as 80% humidity in the room. This means that mould begins to grow on cold component surfaces if the surface is at least cold enough that a moisture level of 80% can be reached in the directly adjacent air layer. The temperature at which this occurs is the so called mould temperature θS. Mould formation thus already begins at temperatures above the dew point temperature.
For an indoor climate of 20 °C and 50% relative humidity, the mould temperature is 12.6 °C (see Figure 32), which means it is 3.3 °C higher than the dew point temperature (see Figure 31). For this reason, the mould temperature is the decisive aspect of preventing structural damage due to mould formation. Surface temperatures must therefore be kept above the mould temperature.
Predicting Surface Temperature
The temperature factor is a parameter that helps evaluate the risk of mould growth and condensation on the inside surfaces of a construction.
The temperature factor fRsi is defined as the temperature difference between the inside surface temperature and the outside air temperature (θsi – θe) divided by to the temperature difference between the inside and outside air (θi – θe):

Minimum requirement according to IP1/06
The purpose of the minimum requirements for the fRsi value is to prevent damage to the structure from condensation. The general rule for dwellings is:

If Accredited Construction Details are used, there is no requirement to prove thermal performance. If another detail is used, numerical modelling must be carried out to prove that fRsi > 0.75
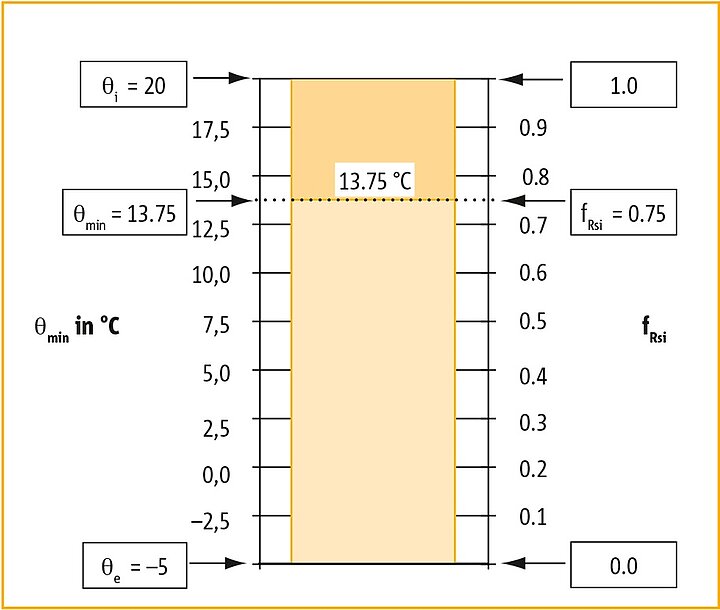
The fRsi is a relative value and thus provides the advantage that it only depends on the design of the thermal bridge and not on the external and internal air temperature like θsi. The value of the temperature factor when f = 1 is if the minimum inside surface temperature θmin is the same as the inside air temperature and when f = 0 is if it is the same as the outside air temperature (see Figure 33).
The minimum surface temperature is determined using three dimensional numerical analysis. With this number and with the air temperature inside and outside it can be calculated thus:

In this example the surface temperature is 15°C and the air temperatures are 20°C and 0°C, this results in an ƒRsi of 0.75.